Laurent Autrique Homepage |
Laris - Université d'Angers | ||||
 |
|||||
| Home | Research projects | Publications | Teaching | ||
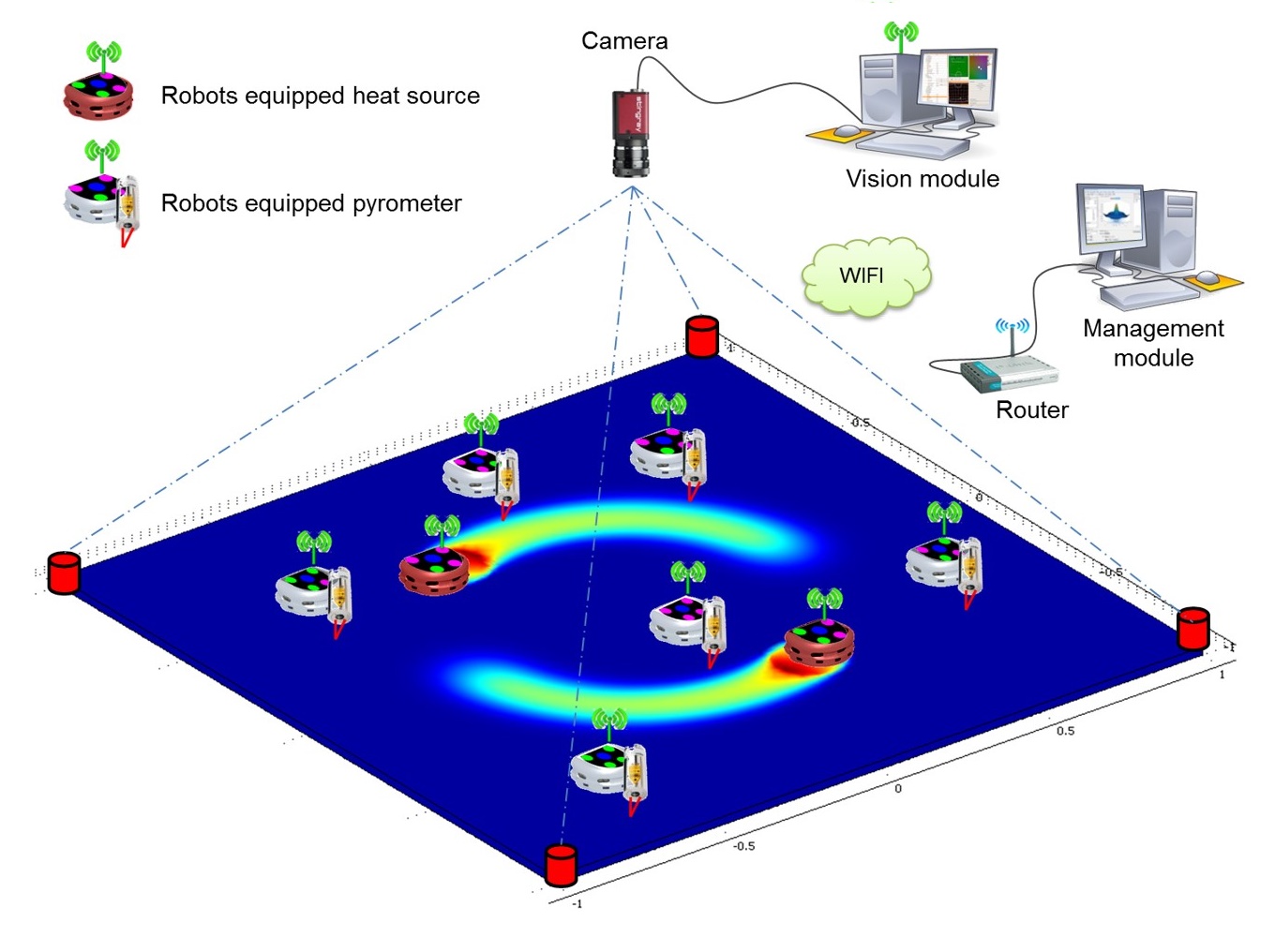Nowadays the environment and people protection requires the use of sensors to monitor the movement of mobile phenomena to predict and act on their evolution (ex: polluting cloud, fires, oil slick). These physical phenomena are often modelled by partial derivative equations (PDE) possibly non-linear. Development of a predictive tool requires the identification of some input parameters. In these areas, the sensors are generally expensive and limited. However, considering recent technological progresses, it becomes possible to develop low-cost mobile systems and to deploy in a proper way a group of networked vehicles in a number of environments at risk. Research topic proposed in this project concerns the development and validation of optimal strategies for moving a set of sensors for the parametric identification of PDE systems characterized by one or more mobile sources. This work is intended to develop a comprehensive methodology (theory, numerical methods, and experimentation) in order to achieve an optimal design of experiments in the context of nonlinear ill-posed problems related to the evaluation of parameters in systems described by PDE in thermal engineering. 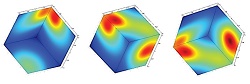 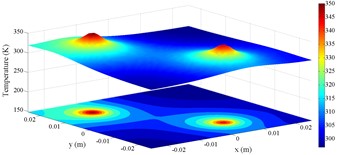 The proposed approach is based on an adaptation of a regularization method: the conjugate gradient method. Such method is widespread in offline context and well known for inverse heat conduction problem. In the studied context, the aim is to reduce computational time in order to obtain identification results on time interval as fast as possible after the current measurement. Then the overall method is developed in the aim of delay reduction under the constraint of a reliable identification.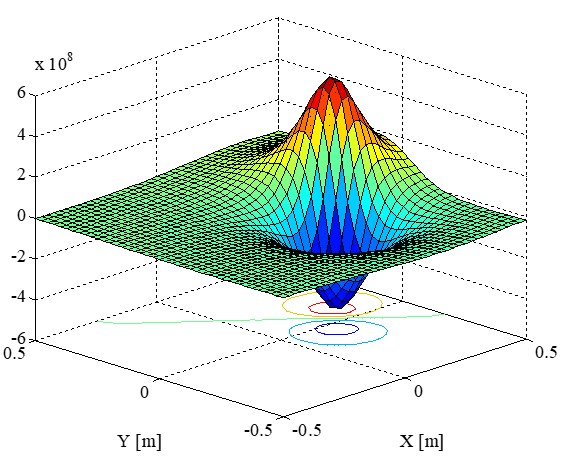 
62 avenue notre dame du lac, 49000 Angers Phone : +33 244 687 518 | laurent.autrique at univ-angers.fr |
CV LARIS CLUB EEA Polytech'Angers 
|